

1. individuals (ie 1, 2)
2. commodities (ie 1, 2, x (x1, x2), p= (p1, p2)
3. preferences of individuals
4. endowment ei = (ei1, ei2)
If we give the p every individual computes its demand xi (p)
To aggregate xa (p)=sum of xi (p)
zi (p)=xi (p)-ei
Aggregate excess demand za (p)=sum z1 (p)=xa (p)-ea
budget line at p (goes through e)
If you have flat segments in indifference curves. You get set as an answer
zi (p)={xi}-{ei}
px=pea
pza=0
![]() 2
2
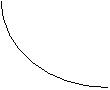 Budget line at p going
through e
Budget line at p going
through e

p*x1< xi(p)
p*ei e
![]() 2
2
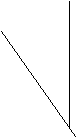
z

p
![]() 0
0
for every p za (p) is convex set. It is continuos and it has the right behaviour as prices tend to the boundary)
When you have nonconvexities, adding more people will allow us to close the gaps. Then an equilibrium in the average will mean an average - some people on both equilibrium.
Continuity - if individuals have strictly positive endowment of each good. Or it also does not occur when nobody is impoverished (value of endowment is 0). Or any division endowment of group one is always >1 for group 2.
1. utility function
2. endowment
3. technology
unit input of coefficient Aij
Aww=280/400
200w+12I=400w
120w+8I=20I
subsistence economy-only one independent equation
Perron Frobenius theorem on nonnegative square matrixes. Only 1 will have a nonnegative solution
commodities that are used to produce others are basics, other are not. Price of non-basics will not change the profit rate.
Data
(neoclassical)
1. utility function
2. Endowment
Data classical
1. structure of production (technique)
If you tax basics it will change the profit rate, if you tax gold, profit remains the same
If you add labour that is not produced, then from Frobenios theorem you get an inverse relationship between wages and profit
(1+r) (a11+a21p2)+al1w=1
(1+r) (a21+a22p2)+al2w=p2
3rd frobenious - if any of the coefficients rise then the rate of profit falls
Growth
1. Capital accumulation
2. technological progress
1. y=f (k, l) constant return